No, really, calculus can be beautiful and this mathematician will tell you why

It's probably been some time since you thought about calculus. But Steven Strogatz thinks maybe it's time you gave the subject another chance.

Whether we encountered calculus last in high school, or possibly early in university, for many it was a somewhat traumatic encounter with a mathematical discipline that can be unforgiving.

But behind the difficulty is hidden an extraordinary power that, according to Strogatz, is responsible for much of our modern life. It lies behind the engineering and flight of airplanes and spacecraft, it helped solve the puzzle of HIV treatment, and is even essential to modern entertainment as it enables the rendering of detailed CGI in movies and video games.

In his new book, Infinite Powers, How Calculus Reveals the Secrets of the Universe, Strogatz, a professor of mathematics at Cornell University in New York, tells these stories and explains why he things "calculus is the language of the universe, as well as it's logical engine."

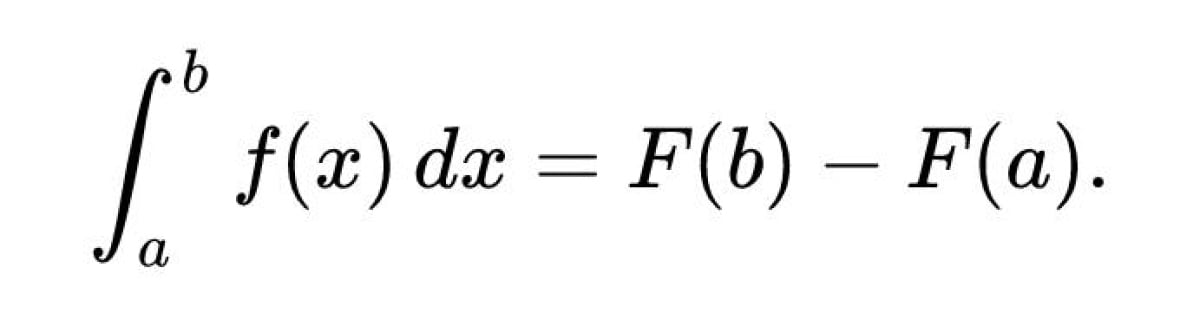
Steven Strogatz spoke to Bob McDonald of CBC's Quirks & Quarks about his new book

This transcript has been edited for length and clarity

Bob McDonald: Let's start at the beginning. What exactly is calculus?

Steven Strogatz: If you had to say it in a word, it's the mathematics of change.

Imagine a ball rolling down a ramp, not the most exciting thing in the world to think about, but what's interesting about it is that it picks up speed as it rolls. There was a time when humanity didn't understand the laws of falling objects and that led to what we nowadays call differential calculus.

Now that doesn't sound like much of a payoff, you can understand how things roll down a ramp. But today we use differential calculus to understand the level of virus in the bloodstream of a patient with HIV. What used to be a near certain death sentence is now a manageable, though chronic, illness and calculus was a big part in figuring out the triple combination therapy that has been a lifesaver for millions of people.

BM: You describe in your book not only how calculus is about change but how it's about curves and figuring out areas. You talk about how infinity turns out to be critical for calculus. Tell me about that and why do you need infinity?

SS: Infinity is a key player in thinking about change of any kind.  When you're dealing with a curved shape, something's changing there. A curve is something that changes direction, if it didn't change direction it would be a straight line. So when you have curves you're talking about a certain kind of change, a change of direction, and that makes the math difficult.

We did a lot of questions in school about triangles and squares and other shapes that are made up of straight lines. But when you have something like a circle or a parabola or any other curve, it's very difficult to do calculations of the area under the curve.

It's an obvious point that a circle is round, it doesn't contain any straight segments. But in calculus we pretend that it does. We pretend that a circle, if you zoomed in on it under a microscope, you'd see that the circle would start to look straighter and straighter under increasing magnification. It's almost as if it were made up of many infinitesimally small straight pieces.

This is the great brainstorm of calculus. Think about an object that's complicated and pretend it's made up of much simpler pieces, at the cost of having to imagine infinitely many of them. So harnessing infinity turned out to be the key to solving lots of problems that were previously intractable.

BM: Then you take that further in your book right up to modern computer graphics which use many little tiny straight lines to make animations.

SS: If you think about Shrek or Toy Story or any of those computer generated movies, what's going on if you dive deep into the computer graphics is that Shrek's round belly is actually made up of millions of polygons triangles and squares. They're so tiny you don't see them, you don't perceive that his belly is faceted, it looks smooth, but in the computer we represent any sort of shape using an enormous aggregation of triangles and other rectal linear shapes.

We've also used the same idea to improve facial surgery, with the help of computer graphics and simulations of the soft tissue of a person's skull. It's with the help of calculus that now surgeons have what is in effect a flight simulator for facial surgery.

BM: Well I get it that if you have one problem that you can't solve, you solve it by making an infinite number of smaller problems. But now you've got an infinite number of problems.

SS: Well the strategy comes with a cost, as you say, that when you divide a hard problem into an infinite number of smaller, and hopefully easier problems, you're still stuck with infinity small problems. Now you've got to put them back together to solve the original problem. That's the other half of calculus, the part we call integral calculus, where you have to integrate all the answers back into an integrated coherent whole. That is the harder part of calculus and the part that certainly our students find more difficult.

Isaac Newton or Gottfried Leibniz, they found a trick that nowadays we call the fundamental theorem of calculus. It's a way of making calculations so much faster and so streamlined that you can now teach them to ordinary high school students.

BM: Well it's hard for those of us who've been out of school for a long time and don't use advanced math as a tool in our everyday lives to really appreciate something like calculus. So what's the one thing you would want people to understand about it?

SS: I would like people to come away understanding what a triumph of human creativity and imagination it is. That it's built on this one vision that we can cut things into finer and finer pieces. By pretending that we can divide everything infinitely finely, it works so well that it has been a revolutionary idea. It has really changed the world.